Chapter: Integration by parts
Q2. Find the integration of `\e^{ax}\cosbx`
Sol: Let ` I = \e^{ax}\cosbx`
From integration by parts method
`\1^{st}part\int {2^{nd}part - } \int {(\frac{d}{{dx}}} {1^{st}}part\int {{2^{nd}}partdx)dx} \]` …………………(1)
So, let `1^{st}part = \e^{ax}` And `2^{nd}part = cosbx`
Now put these values in eq (1) we get-
` I =\int\e^{ax}\cosbx`
I = `e^{ax}\int \cos bx -- \int (\frac{d}{dx} e^{ax}\int \cos bxdx)dx `
I = ` \frac{1}{b}e^{ax}.\sinbx - \int a e^{ax}\frac{\sinbx}{b}dx`
I = `\frac{1}{b}e^{ax}.\sinbx - \frac{a}{b}[e^{ax}\int \sinbxdx - \int (\frac{d}{dx} e^{ax}\int \sinbxdx)dx]`
I = ` \frac{1}{b}e^{ax}.\sinbx - \frac{a}{b}[-e^{ax}\frac{\cosbx}{b} + a\int {e^{ax}.\frac{\cosbx}{b}dx} ]`
I = `\frac{1}{b}e^{ax}.\sinbx + \frac{a}{b^{2}}e^{ax}\cosbx` - `\frac{a^{2}}{b^{2}}\int e^{ax}.\cosbxdx `
I
I + `\frac{a^{2}}{b^{2}}.I`= `\frac{1}{b}e^{ax}.\sinbx + \frac{a}{b^{2}}e^{ax}\cosbx`
I.(`\frac{a^{2}+b^{2}}{b^{2}})` = `e^{ax}. \frac{b.sinbx + a.cosbx}{b^{2}}`
Or I = `e^{ax}. [\frac{b.sinbx + a.cosbx}{a^{2}+b^{2}}]`
Which is our final answer for the integration of `\e^{ax}\cosbx`
Similarly we will also find integration of `\e^{ax}\sinbx`
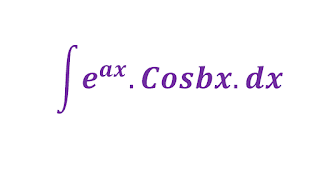


No comments:
Post a Comment