Integration - By Partial Fraction
*Please rotate your mobile screen for better view
*Integration of `\int \frac{dx}{x^2 - 4}`
Sol: Given I = `\int \frac{dx}{x^2 - 4}`
Let ` \frac{1}{x^2 - 4}` = ` \frac{1}{(x - 2)(x +2)}`
Now decomposing in partial fractions
` \frac{1}{(x - 2)(x +2)}` = ` \frac{A}{x - 2}` +` \frac{B}{x +2}` .....................(1)
= ` \frac{A.(x+2) + B.(x-2)}{x^2 - 4}`
OR = ` \frac{(A+B).x +(2A-2B)}{x^2 - 4}`
Now Comparing coefficients of 'x' and constants on both sides of equal sign, we get
A+B = 0 i.e., A = -B ...............(2)
& 2A - 2B = 1 ............(3)
Now putting the value of ' A' from equation (2) to equation (3) we get.
-2B - 2B = 1
OR -4B = 1 => B = - ` \frac{1}{4}`
& from the value of 'B ' we get 'A' as: A = ` \frac{1}{4}`
Now substituting these values in equation (1) we get.
` \frac{1}{(x - 2)(x +2)}` = `\frac{1}{4} \frac{1}{x - 2}` - ` \frac{1}{4} \frac{1}{x +2}`
Now performing integration on both sides w.r.t x.
= `\frac{1}{4}\int \frac{dx}{x - 2}` - ` \frac{1}{4} \int\frac{dx}{x +2}`
= `\frac{1}{4}.log(x - 2)` - ` \frac{1}{4}.log(x + 2)` + c
OR I = `\frac{1}{4}.log\frac{x - 2}{x + 2} ` + c
See Also:
1. Integration of `\e^{ax}\cosbx` - By Parts Method
2. Integration of `e^(ax) sinbx` - By Parts Method
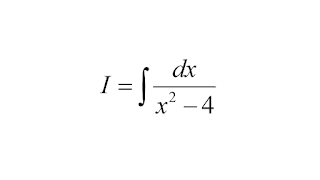


Hey kindly solve more questions like this
ReplyDeleteKindly solve more questions like this
ReplyDelete